Research
I have done research in algebraic number theory. My main interests were the p–adic theory of modular forms, elliptic curves and p–adic Hodge theory.
More information on my works and download links are provided below.

Iwasawa theory and p–adic Hodge theory for relative Lubin–Tate extensions
Personal notes for original work that I have not published formally. Its main contribution is the generalization to the relative Lubin–Tate case of recent research towards a version of Iwasawa theory for (classical) Lubin–Tate extensions.
This work adapts the proofs from articles by (subsets of) L. Berger, L. Fourquaux, M. Kisin, W. Ren, E. de Shalit, P. Schneider, J. Teitelbaum, O. Venjakob and B. Xie.
These notes explain the construction of a p–adic regulator map from a group of Iwasawa cohomology with respect to a relative Lubin–Tate tower of extensions to a space of distributions and work towards an interpolation formula.
The study of p–adic representations of the Galois group of extensions over is complicated because there are many of them. One of the key ideas of p–adic Hodge theory is to shift the difficulty from the modules to the base rings: nice classes of such representations can be embedded into categories in the realm of (semi)linear algebra over what are called period rings. The definitions of these base rings depend on the theory of fields of norms for a totally ramified extension with certain restrictions. The simplest and most studied case is that of the cyclotomic extension, but a theory for relative Lubin–Tate extensions is possible too.
In this more general setting, the work of M. Kisin and W. Ren (based on fundamental results of J.-M. Fontaine) provides equivalences between categories of (crystalline) representations and of (analytic) –modules over period rings. Moreover, the theory of character varieties started by P. Schneider and J. Teitelbaum allows one to relate locally analytic distributions to elements of period rings. With these ingredients and several notions of duality, P. Schneider and O. Venjakob found a way to construct distributions from Iwasawa cohomology classes. An interpolation formula for the regulator map defined in this way can be established by comparing it with a big logarithm map defined by L. Berger and L. Fourquaux that goes in the opposite direction.
The passage from classical Lubin–Tate extensions to relative Lubin–Tate extensions (as defined by E. de Shalit), which is the main contribution in these notes, is mostly of technical nature. Proofs become more involved but the central ideas remain largely unaltered.
@unpublished{gispert2022iwasawa,
author={Gispert, Francesc},
title={Iwasawa theory and \(p\)--adic Hodge theory for relative Lubin--Tate extensions},
date={2022-01-31},
pagetotal={188},
note={Unfinished personal notes},
url={https://fgispert.cat/recerca.html}
}
Andreatta–Iovita’s p–adic L–functions
Study notes for a seminar organized by Ju-Feng Wu and myself. They contain new more detailed explanations of (parts of) F. Andreatta and A. Iovita’s admittedly difficult-to-read article Triple product p–adic L–functions associated to finite slope p–adic families of modular forms.
These notes present the geometric construction, due to F. Andreatta and A. Iovita, of p-adic families of overconvergent sheaves of modular forms and the usual operators acting on them.
There are two important obstacles to generalizing certain constructions of p–adic L–functions attached to ordinary modular forms to the case of modular forms of finite slope:
- There is no analogue of Hida’s ordinary projector because the U operator is not compact on p–adic modular forms. One then needs to view modular forms of finite slope as overconvergent sections of certain modular sheaves.
- It is not clear at all what the analogue of p–adic iterates of the Maass–Shimura operator should be. For one thing, the unit-root splitting of de Rham cohomology only works over the ordinary locus. More importantly, one needs to control the reduction in overconvergence arising from the p–adic iterates.
Formal vector bundles with marked sections are a modification of usual vector bundles with additional congruence conditions. Their construction is functorial and preserves extra structure like filtrations and connections. Applying it to certain modular and de Rham sheaves produces huge sheaves of sections containing all p–adic interpolations of modular forms and of de Rham cohomology, respectively.
Thanks to the geometric nature of the constructions, Hecke operators and twists by characters with the desired properties can be defined. In addition, one can do calculations locally to control the powers of p that arise from iterations of the Gauss–Manin connection.
@unpublished{gispert2020lfunctions,
author={Gispert, Francesc},
title={Andreatta--Iovita's \(p\)--adic \(L\)--functions},
date={2020-03-13},
pagetotal={49},
note={Unfinished seminar notes},
url={https://fgispert.cat/recerca.html}
}
Modular forms modulo p
Master’s thesis for the Algant degree programme, submitted to Universität Regensburg and Università degli Studi di Padova. It contains a detailed presentation of several aspects of Katz’s geometric theory of modular forms that are obscure or miss proofs in the literature.
Abstract | Bib | Thesis | Presentation slides
This thesis presents the geometric theory of modular forms in positive characteristic p, mainly due to N. Katz.
Under certain hypotheses, there are modular curves parametrizing elliptic curves with additional level structures of arithmetic interest. Katz defined modular forms as global sections of a family of line bundles over these modular curves. The tools provided by modern algebraic geometry make the constructions of the curves and line bundles work essentially over any base ring.
Moreover, the modular curves have a special kind of points called the cusps. The cusps parametrize a kind of degenerate elliptic curves known as Tate elliptic curves. The germs of a modular form at the cusps correspond to power series in one variable called the q–expansions of the modular form. The q–expansions often hold a lot of information about the modular form.
In particular, over an algebraically closed field of characteristic p, the algebra of modular forms has a very simple description in terms of a modular form known as the Hasse invariant. Specifically, modular forms are determined up to powers of the Hasse invariant by their q–expansions. In addition, there is a differential operator acting on the algebra of modular forms which helps us to understand this structure.
@thesis{gispert2018modformsmodp,
author={Gispert, Francesc},
title={Modular forms modulo \(p\)},
date={2018-07-16},
institution={Universität Regensburg and Università degli Studi di Padova},
type={mathesis},
pagetotal={99}
}
Modular symbols
Bachelor’s thesis for the degrees in mathematics and in informatics engineering, submitted to Universitat Politècnica de Catalunya. Its main contribution is an explicit proof of the relationship between theory and computations with modular symbols, which is always taken for granted in the literature.
Abstract | Bib | Thesis | Presentation slides
This thesis presents the classical theory of modular forms and modular symbols as well as the links between theory and computation of modular symbols.
Modular forms are holomorphic functions defined on the complex upper half-plane which transform in a certain way under the action of some group of matrices. The orbit space of this action on the upper half-plane admits a structure of Riemann surface and so is called a modular curve. The spaces of modular forms are finite-dimensional complex vector spaces which can be identified with certain spaces of differential forms on the corresponding modular curve.
There is a very important family of operators acting on the space of modular forms, the Hecke operators. One of their main properties is that there exist bases of modular forms consisting of eigenvectors of most Hecke operators; these modular forms are known as eigenforms.
Finally, modular symbols can be thought of as formal symbols satisfying certain algebraic relations and which provide a simple way to represent elements of the first homology group of modular curves (regarded as compact surfaces). The pairing given by integration of a form along a path provides a duality between modular forms and modular symbols. Therefore, Hecke operators also act on the space of modular symbols. One can recover information about the modular forms from the action of Hecke operators on the modular symbols. In conclusion, modular symbols constitute an appropriate setting to perform computations with modular forms and Hecke operators.
@thesis{gispert2016modsymbols,
author={Gispert, Francesc},
title={Modular symbols},
date={2016-04-24},
institution={Universitat Politècnica de Catalunya},
type={bathesis},
pagetotal={123}
}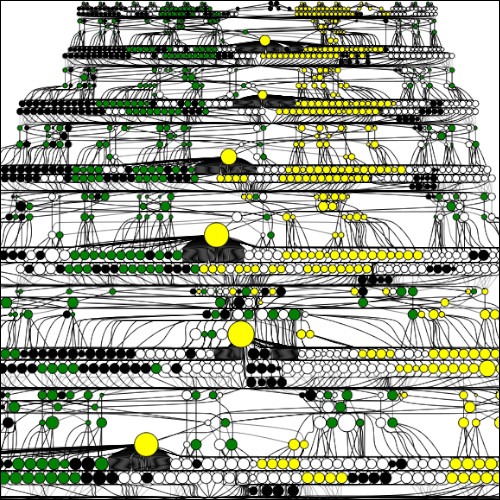
An analysis of the data dependency graphs of k–means
Internal report of a summer internship inside the RoMoL team at the Barcelona Supercomputing Center. It is an experimental analysis of data transfer during the execution of a parallel application depending on the strategy to allocate tasks. It was meant to justify or dismiss further work in this direction.
Abstract | Bib | Report | Presentation slides
This document is the report of an experimental analysis of the impact of graph partitioning techniques, applied to data dependency graphs, in minimizing data transmission in parallel applications.
High-performance computing applications have to carefully adapt their behaviour to the underlying architecture. For instance, the different tasks which an application is divided in can be mapped to the processing units using several approaches. The way this mapping is carried out has a huge impact on the load balance of the cores and the communication between them.
A parallel application can be represented with a data dependency weighted directed graph whose nodes are the tasks and whose edges represent the data dependencies between tasks. I present some graph partitioning techniques which can be applied to the data dependency graphs of parallel applications in order to obtain partitions with little edge-cut.
I analysed and compared experimental results on graphs produced by a concrete application, the k–means algorithm implemented in OmpSs, in order to show that the use of this partitioning step may have a significant impact on performance due to the heavy decrease in communication volume. In particular, the METIS algorithm produced almost optimal partitions, as can be observed from the simple structure of the studied code.
@report{gispert2014partitioning,
author={Gispert, Francesc},
title={An analysis of the data dependency graphs of \(k\)--means},
date={2014-09-09},
type={Internship report},
institution={Barcelona Supercomputing Center},
pagetotal={16},
note={Internal unpublished document}
}